Renovation at the basement gambling den

The foundations of probability theory were reset by Kolmogorov in the 1930s. Until then, they'd rested on the relative frequencies of outcomes of randomisation machines.  The new set of axioms made no mention of randomisation machines or experiments or gambling.  Instead they tied a set of numbers to a second set, of events, no need to say any more than that.  The first two axioms constrain the numbers, the third associates these numbers with the internal relationships of the reference set of events.  For any good reference set of objects, each set of numbers which satisfied the axioms could be called a probability distribution with respect to the reference set $E$.

1.  $\forall A \in E,  P(A) \geq 0$
2. $P(E)=1$
3. $P(\bigcup_{k=1}^{\infty}A_k) = \sum_{k=1}^{\infty}P(A_k)$ for each and every infinite sequence of disjoint events $A_k$

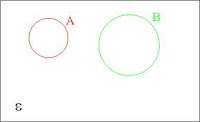
Axiom 3 is doing the work.  The idea of disjoint events is from set theory.  The following shows two disjoint events.

 And here's a pair of events which are not disjoint.

While this isn't the whole story on independence and mutual exclusivity, I won't go further on it at this point.

Axioms 1 and 2 allow you to synthesise some of the phenomena of relative frequencies without having to mention them - namely that, since a relative frequency is a ratio of one count over a second, larger, all encompassing count, it will always lie somewhere between 0 and 1.  Kolmogorov, after all, is re-modelling the foundations, not pulling down the entire edifice.

Axiom 3 is all about relationships.  This set of numbers, this probability distribution, clearly can't just be any set of numbers.  Some of those numbers relate to each other in a very specific way.  Axiom 3 states that there's a summation relationship (actually infinitely many of them) between a number and a bunch of other numbers precisely insofar as their referent events, which, remember are disjoint, when $\bigcup$-ed together equal each other.

Spelling it out for just one case.  Imagine there is an infinite set of disjoint events $e_i$.  Performed a set union on them all let's call the resulting set $E_i$.  $\forall e_i$ and the single $E_i$ both are already in your reference set.  Now that we have identified this set relationship between  $\forall e_i$ and the single $E_i$ we  make a corresponding claim about the set of numbers that constitute this particular probability distribution.  Let's call that set of numbers $n_1, n_2, ..., n_i$ and the single number $N_i$.  Axiom 3 tells us that we can be certain that $n_1 + n_2 + ... + n_i = N_i$

This isn't a moral point about gambling or even empiricism.  If anything it is motivated by an aesthetic-mathematical impulse.